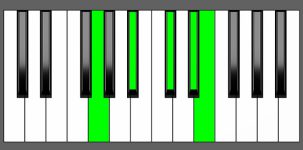
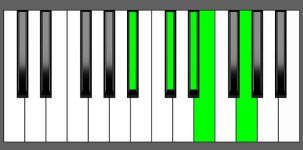
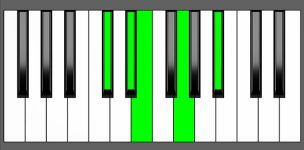
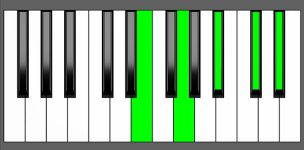
Piano Diagram of D#7b9 in Root Position

A D#7b9 chord is an altered dominant seventh chord that is built upon the key of D#. This chord consists of the root D#, the major third Fx, the perfect fifth A#, the minor seventh C#, and the minor ninth E. The D#7b9 chord can be used as a substitute for dominant chords only in specific musical contexts. Keep reading to learn more about the music theory that underpins this chord.
Structure of D#7b9
Notes |
|---|
| D#, Fx, A#, C#, E |
Intervals |
|---|
| R, 3, 5, m7, m9 |
How to play a D#7b9
To play a D#7b9 chord, you can use the following voicing: start by playing the root note D# with your left hand. Then, with your right hand, play the notes Fx (G, major 3rd), C# (minor 7th), and E (flat 9th).
D# + Fx, C#, E
This approach will result in a simplified D#7b9 chord that includes only the essential notes: the root note, major 3rd, minor 7th, and minor 9th.
D#7b9 Chord Equivalencies
When you remove the root note from a 7b9 chord, it becomes a diminished 7th chord. In the case of a D#7b9 chord, if you remove the root note D#, you’re left with the notes Fx, A#, C#, and E, which are equivalent to an Fxdim7 chord (Gdim7).
D#7b9 without root = Gdim7
Gdim7 = Bbdim7 = Dbdim7 = Fbdim7
Diminished 7th chords have a unique quality where each inversion is another diminished 7th chord. So, Gdim7 is enharmonically equivalent to Bbdim7, Dbdim7, and Fbdim7 (Edim7). Therefore, even if you remove the root note from a D#7b9 chord, it is still enharmonically equivalent to all of these chords.
D#7b9 Chord Inversions
The D#7b9 chord has a total of 4 inversions:
| Root Position: | D# | Fx | A# | C# | E |
| 1st Inversion: | Fx | A# | C# | D# | E |
| 2nd Inversion: | A# | C# | D# | E | Fx |
| 3rd Inversion: | C# | D# | E | Fx | A# |
| 4th Inversion: | E | Fx | A# | C# | D# |
Piano Keyboard Diagrams
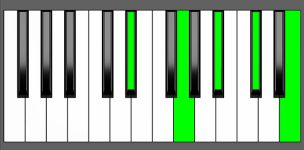
Chord Inversions on Piano
Understanding chord inversions is crucial for music theory, as it reveals how chords are constructed. When playing chord inversions on a piano, it’s important to remember that the charts showing the order of notes may not always be playable.
To properly voice chords on a piano, the notes must be spread across various octaves and positions on the keyboard. This often means deviating from the standard shape of the chord’s inversions, as shown in charts. It’s always a good idea to experiment with different voicings and fingerings to find the most efficient and comfortable way to play the chord, while still maintaining its intended harmonic function and sound.
Chord inversion charts can help understand the structure and sequence of notes in a chord, but it’s important to be flexible and adaptable when playing them on the piano. With practice, you can find your own unique voicings and develop a personal style that suits your playing needs.
Music Theory and Harmony of D#7b9
The D#7b9 chord can replace or enhance the D#7 chord, typically on the V degree, but also on the III degree, and occasionally on other degrees as a secondary dominant chord. However, it has a distinct vibe, so it should be used carefully in the appropriate musical context.
Before delving into the most frequent uses of this chord, let’s first understand how to build it.
Building the D#7b9 Chord: Different Approaches
Starting from the D# Major Scale:
To form a 7b9 chord, you combine the root, the major 3rd, the 5th, the minor 7th, and the flat 9th from a major scale.
To build a D#7b9, you can start with the D# Major scale:


To create a D#7b9 chord, apply the formula R, 3, 5, m7, m9 in the following manner:
- Begin with the Root note, which is D#.
- Select the major 3rd interval, which is Fx, and add it to the chord.
- Add the 5th interval, which is A#.
- Add the minor 7th interval, C# (Cx less a half tone).
- Finally, select the 9th E# and subtract a half tone to get the minor 9th, E.
By following this simple formula, you can create a 7b9 chord from any major scale.
by Combining Intervals:
One method to create a 7b9 chord is by combining specific intervals – a major 3rd, a minor 3rd, and another minor 3rd.
3 + m3 + m3 + m3 = 7b9 chords
For example, to build a D#7b9 chord:
- we start with the root note D#.
- We then add a major 3rd interval, which is four half-steps up from the root, to get G (Fx).
- Next, we add a minor 3rd interval, which is three half-steps up from Fx(G), to get A#.
- Then, we add another minor 3rd so we find the minor 7th C#
- and lastly, we add a minor 3rd interval from C#, to get E.
Together, these intervals form the D#7b9 chord.
by Combining Chords
Another way to build a 7b9 chord is by combining a major triad with a diminished triad derived from its 5th.
To build a D#7b9 chord, you can blend a D# Major triad (D#, Fx, A#) with an A# diminished chord (A#, C#, E). The A# note is shared between the two chords.
D# Major + A# dim = D#7b9
How to Use D#7b9 in a Chord Progression
The D#7b9 can work as a substitute or as a passing chord to a D#7 that can be found in major and natural minor scales. The 7b9 chord is considered a non-diatonic chord, which means that it contains notes that are not found in either the major or minor scales.
Most common uses of D#7b9
D#7b9 in G# Major and G# minor
The D#7b9 chord is commonly used as a dominant chord. In the key of G# major, the D#7b9 chord can be used as the V7 chord, which leads back to the I chord (G# major).
However, since G# major is a theoretical key, it’s better to refer to its enharmonic equivalent key Ab.
| Major Scale | I | ii | iii | IV | V | vi | vii |
|---|---|---|---|---|---|---|---|
| Ab = G# | Ab Maj7 | Bb min7 | C min7 | Db Maj7 | Eb7 ⇒ Eb7b9 = D#7b9 | F min7 | Gm7b5 |
- Substitute or Passing Chord to the Dominant chord in Ab Major as Eb7b9
D#7b9 as Substitute of D#m7
The D#7 chord derived from the harmonic minor scale is commonly used to replace a D#m7 chord in the key of G# minor. In some cases, the D#7b9 chord can also be used instead of the D#7 chord, further enhancing the harmonic tension and leading to the G#m7 chord.
| Minor Scale | i | ii | III | iv | v | VI | VII |
|---|---|---|---|---|---|---|---|
| G# | G# min7 | A#m7b5 | B Maj7 | C# min7 | D#m7 ⇒ D#7 ⇒ D#7b9 | E Maj7 | F#7 |
- Substitute or Passing Chord to the Dominant chord in G# minor
D#7b9 in a I – III7 Progression
The D#7b9 chord is often used as a substitution for the III degree in an I – III7 chord progression, which traditionally features a D# minor chord. This chord progression, represented by the chords B Maj7 and D#7, can accommodate the D#7b9 chord in place of the expected D# minor chord. The D#7b9 chord provides a dissonant augmented triad that offers a unique flavor to the progression.
| Major Scale | I | ii | iii | IV | V | vi | vii |
|---|---|---|---|---|---|---|---|
| B | B Maj7 | C# min7 | D#m7 ⇒ D#7 ⇒ D#7b9 | E Maj7 | F#7 | G# min7 | A#m7b5 |
- Substitute or Passing Chord to the Mediant chord in B Major
D#7b9 in E# minor
While the D#7b9 chord can be used in the key of E# minor, it may not be the most common use of this chord. The resolution to E# minor may be less strong than G# major or G# minor.
Even in this case, we will refer to its enharmonic equivalent key F minor.
| Minor Scale | i | ii | III | iv | v | VI | VII |
|---|---|---|---|---|---|---|---|
| E# = F | F min7 | Gm7b5 | Ab Maj7 | Bb min7 | C min7 | Db Maj7 | Eb7 ⇒ Eb7b9 = D#7b9 |
- Substitute or Passing Chord to the Leading Tone chord in F minor as Eb7b9 (less common)
D#7b9 as Substitute of a Secondary Dominant 7th chord
A secondary dominant is a chord that doesn’t belong to the main key of a musical piece but is used to create a strong pull toward another chord that does. In Western music, the fifth scale degree has a dominant function and generates tension that resolves to the first scale degree chord (I). A secondary dominant chord is used to create the same dominant function but towards a different chord, resulting in a brief departure from the main key.
For instance, in the key of C# major, G#7 is the V chord and resolves to the I chord (C# Maj7). To create a secondary dominant, another chord is added between C# Maj7 and G#7, which creates a powerful pull towards G#7. In this scenario, incorporating a D#7 generates a pull towards G#7 since D#7 is the V chord in the key of G#.
| C# Maj7 | G#7 |
⇒
| C# Maj7 | D#7 | G#7 |
Instead of using a regular D#7 chord, you can also use the D#7b9 chord in place of or together with it. This substitution or addition can add more tension and complexity to the progression, leading to a more interesting and dynamic musical result.
| C# Maj7 | G#7 |
⇒
| C# Maj7 | D#7/D#7b9 | G#7 |
D#7b9 as Dominant Chord in G# Major
D#7b9 as Dominant Chord in G# minor
In the key of G# major, the D#7 chord can be found on the V degree of the G# melodic and harmonic minor scales. This chord is frequently used in combination with chords built on the natural minor scale, or it can even replace the D#m7 chord. In certain musical contexts, a D#7b9 chord can be used as a substitute for the D#7 chord in this position, adding a unique and complex sound to the music.
| i | ii | III | iv | v | VI | VII |
| G# min7 | A#m7b5 | B Maj7 | C# min7 | D# min7 ⇒ D#7 ⇒ D#7b9 | E Maj7 | F#7 |
D#7b9 as V degree on Minor Scale – Chord Progressions
Try playing these chord progressions to get an idea of how D#7b9 functions as the subdominant (V degree) in the key of G# minor.
ii V7 i
| ii | V7 | i |
| A#m7b5 | D#7#9 | D#7b9
D# (Fx, C#, F#) | D# (Fx, C#, E) |
G# min7 |
i iv V7
| i | iv | V7 |
| G# min7 | C# min7 | D#7b9 | D#7 |
i iv VI V7
| i | iv | VI | V7 |
| G# min7 | C# min7 | E Maj7 | D#7#9 | D#7b9 |
Circle Progression
| i | iv | VII | III | VI | ii | V7 | i |
| G# min7 | C# min7 | F#7 | B Maj7 | E Maj7 | A#m7b5 | D#7b9 | D#7 | G# min7 |
D#7b9 as III7 Degree in B Major
The D#7b9 chord can also be used as a variation for the iii chord in the key of B major. This is a common substitution in music where a dominant chord is used instead of a minor chord. In this case, the D#7b9 chord is played in place of the minor chord on the iii degree of the B major scale.
| I | ii | iii | IV | V | vi | vii |
| B Maj7 | C# min7 | D# min7 | E Maj7 | F#7 | G# min7 | A#m7b5 |
D#7b9 as III7 degree – Chord Progressions
Try playing these chord progressions to get an idea of how D#7b9 functions in this position. To understand why this substitution is possible, I suggest checking out some of the posts related to dominant 7th chords. There are different interpretations of this substitution, so it can be helpful to explore them to fully understand its potential use.
I III7 vi
| I | III7 | vi |
| B Maj7 | D#7b9 | D#7 | G# min7 |
I III7 VI7 ii
| I | III7 | VI7 | ii |
| B Maj7 | D#7b9 | D#7 | G#7 | C# min7 |
I III7 IV VI7 ii V iii/biiiø ii/V
| I | III7 | IV | VI7 | ii | V | iii/biiiø | ii/V |
| B Maj7 | D#7b9 | D#7 | E Maj7 | G#7 | C# min7 | F#7 | D# min7 | D dim7 | C# min7 | F#7 |
“All of me” Progression
| I | III7 | VI7 | ii | III7 | vi | II7 | ii/V |
| B Maj7 | D#7b9 | D#7 | G#7 | C# min7 | D#7b9 | D#7 | G# min7 | C#7 | C# min7 | F#7 |
D#7b9 as Leading Tone Chord in E# minor
Alternative D#7b9 Nomenclature
- D# 7b9
- D# 7/b9
- D# 7(b9)
- Re# 7/b9
- D# dom7b9
- D# 7th b9th
- D# 7th flat 9th
- D# Dominant 7th b9
- D# Dominant Seventh Flat Ninth
Conclusion
The chord progressions and examples presented in this post provide a comprehensive overview of the most common uses of the D#7b9 chord. It’s important to note, however, that many advanced harmony-related topics could not be included due to space constraints. These topics include chord progressions built on harmonic and melodic scales, modal scales, hidden tonality, secondary dominants and other chord substitutions, non-functional harmony and atonal music, modal interchange and borrowed chords, voice leading and counterpoint, chromatisms, jazz harmony…I mean, music theory is a huge topic!
Although I couldn’t cover all of these topics in my post, I encourage readers to continue exploring these areas in their own study and research. By expanding your knowledge in these advanced areas of music theory, you can gain a deeper understanding of the harmonic possibilities that exist beyond the basics presented here.